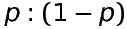Theorem 1
If A and B are events associated with a random experiment having sample space S and if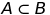 
, then
lessthanequalP(B).png)
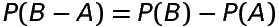
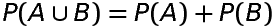If 
are mutually exclusive events associated with a random experiment, then
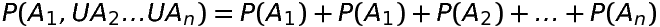
Theorem 2
If A and B are mutually exclusive and exhausive events associated with a random experiment having sample space S, then
are mutually exclusive and exhaustive events associated with
random experiment having sample space S, then
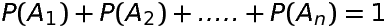
Theorem 3
If A and B are any two events associated with a random experiment having sample space S, then
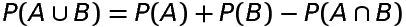
Theorem 4
If A and B are any two events associated with a random experiment, then
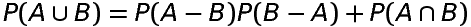
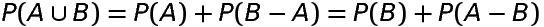
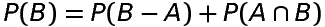
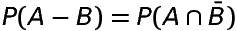Probability of occurence of A only
Probability of occurence of B only
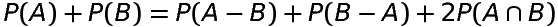
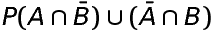is the probability of occurence
of only (or exactly) one of the two events A and B.
Theorem 5
If A, B and C are any three events associated with a random experiment, then
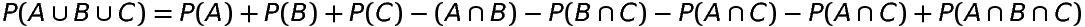
Other Important Properties
If odds in favour of an event A are a:b then
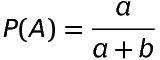If odds against an event A are a:b then
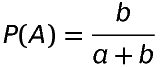If probability of an event is p then odds in favour are
